precisioninfo.com - Dr Bryan Hall - pre 2010
The precision of a record of observation is related to the extent to which the record specifies that the phenomena being observed exists within some abstract interval. Accuracy can be defined by the distribution of record errors. Resolution sets a lower bound on accuracy and it is generally considered good practise to ensure recorded precision falls within limits set by attainable accuracy.
Digital databases can specify locations and report data very precisely and this can create false illusions of data accuracy. Wolf (1993) confirmed that it is important to record all significant figures in measurement. Failing to record the last significant digit is a waste of the extra time and resources spent in obtaining the added accuracy implied by the extra digit. Conversely, when more than the number of significant figures is recorded, a misleading and false level of accuracy is implied.
When information is not sufficient to permit an exact reconstruction of an entity (always), it is impossible to separate the constructed image from the series of instructions that created it. In any data processing operation conservation of information is the best outcome that can be achieved.
All reconstructions that can be put into digital format must reconstruct by some sort of numerical method. It is essential to establish control procedures in any technical reconstruction operation. It is impossible for the accuracy of a finished product to be any better than the control standards on which it is based. If the correspondence between reality and the numerical method's estimate is unsatisfactory then either:
Consider a measuring rod of unit length on which N equal spaced intervals are defined. In order to observe a change, Dt, in a measured variable, it is necessary that t and t+Dt lie on opposite sides of a division on the measuring rule. In order to guarantee that Dt be observable it is necessary that Dt >1/N. This statement asserts nothing more than if it is desired to measure with an accuracy of 1 part in N then there must exist at least N distinguishable states (Resnikoff; 1989). No numerical estimation method can compensate for or correct a problem due to inadequate information. Therefore, before embarking on any reconstruction operation it is necessary to consider the information available.
Information must be structured in a coherent way and it is possible to extract useful information from data only when both the structure of the information being sought and the structure of the data from which the information must be extracted are understood. There are two distinct kinds of information theory that are of concern here. The first is statistical information theory which is concerned with what happens over a long series of uncertain situations where information must be transmitted through some sort of communication channel. In 1948 Shannon published two papers on "A Mathematical Theory of Communication" in the Bell Systems Technical Journal and these papers laid the foundation for the mathematical theory of information. Semantic information theory addresses what we actually mean by the symbols we invoke to communicate (Hintikka; 1970).
Simulations can take many forms and may include any of the following:
Land surface reconstruction algorithms may calculate
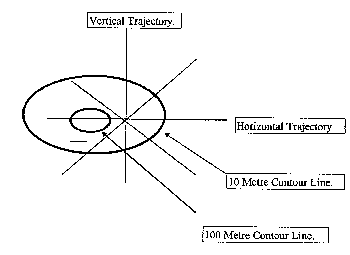
If one had to choose between the linear height estimate provided by the vertical trajectory and that provided by the horizontal trajectory, at the intersection of the two trajectories, then the most reasonable guess would be that provided by the horizontal trajectory.
A linear interpolation between the 100 metre and 10 metre elevation values along the horizontal trajectory would estimate the height at the intersection point as being approximately 50 metres.
The height could also be estimated as 20 metres by evaluating an estimate from each trajectory ((10+10+10+50)/4 metres). Such a method fails to take into account the greater information content of the horizontal trajectory. Such a method will tend to "average out" all the sharp changes in slope and elevation and in so doing "flatten the database". The application of a simple arithmetic average in a situation such as this is mistaken.

This figure shows a slope profile calculated by a reconstruction algorithm that incorrectly applied a simple arithmetic average when calculating a land surface from circular contour information.
Least squares functions and orthogonal projections are generally used to solve equations that are inconsistent only in very limited circumstances (Hill; 1986). A least squares approach assumes that the error or variability in measuring the dependent variable is minimal in comparison to the independent variable. The assumption of linearity can not be sustained under circumstances where it is required to reconstruct a land surface from contour lines.
Any reconstruction algorithm can be tricked and it is to an extent true that all reconstruction algorithms have some directional instability associated with them. Whilst it is not possible to reconstruct objectively from incomplete information, any good reconstruction algorithm would aim to reconstruct consistently.
An algorithm that fails a symmetric test, such as the one given, is not reconstructing consistently and unfortunately the slope and elevation information calculated is dependent on the declaration of north.
Further Information on Land Surface Reconstruction:
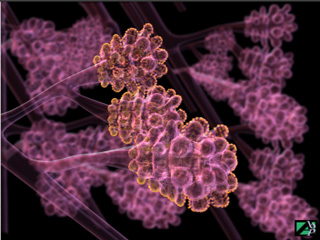
This static image, taken from a medical animation, shows the finest branches of the airway, the respiratory bronchioles, and the alveolar sacs which are involved in gas exchange. The inhalation of irritant fibers of asbestos sets up inflammation which may cause impairment of uptake of oxygen, and can lead eventually to respiratory failure.
People who are exposed to inhalation of asbestos fibers are at risk of developing major pathological changes within the respiratory system. These may be in the form of
These in turn may lead to the development of pleural mesothelioma, a particularly vicious form of malignant disease.
Now that the dangers of asbestos inhalation are widely known, employers must minimise this risk to their workers, by substitution for asbestos using other materials as far as possible, providing protective clothing and masks, and improving ventilation in the workplace
Further Information on Medical Simulations:
References: